Specific heat of condensation is the heat energy to be released for condensation of a gas per kilogram of the substance!
Vaporization and condensation
In the article on specific latent heat of vaporization, it was explained in detail that when a liquid is to be vaporized, energy is required to break the bonds so that the substance changes to the gaseous phase. This energy is added to the substance as heat and is called heat of vaporization. In the case of pure substances, the temperature remains constant during vaporization.

If the gaseous substance is to be liquefied, then the heat previously added must be released so that the molecules can bind to each other again. In this case, the heat energy to be given off during the condensation of the gas is called heat of condensation. Even while the heat of condensation is being released, the temperature remains constant. It only drops again when the gaseous substance has completely condensed. The temperature at which a substance condenses corresponds to the boiling point of the substance.
For a more detailed explanation of the molecular processes that occur during condensation and the reason why the temperature remains constant, see article Why does the temperature remain constant during a change of state (phase transition)?
Specific heat of vaporization and condensation
Due to the conservation of energy, the amount of heat absorbed by a substance to vaporize (heat of vaporization) is equal to the amount of heat released by the substance during condensation (heat of condensation). For this reason, the specific heat of condensation qc, as the ratio of the heat of condensation Qc and the condensing mass mc, is as great as the specific heat of vaporization qv.
\begin{align}
&\boxed{q_\text{c} = \frac{Q_\text{c}}{m_\text{c}}}~~~\text{where}~~~\boxed{q_\text{c} = q_\text{v}} \\[5px]
\end{align}

The specific heat of condensation depends on the substance and indirectly on the pressure, since the boiling point (condensation point) is influenced by the pressure. In the article Specific latent heat of vaporization, the specific heats of vaporization or condensation are listed for selected substances. Using these values, the heat of condensation Qc to be released for a given mass mc to be condensed can be determined with the following formula:
\begin{align}
&\boxed{Q_\text{c} = m_\text{c} \cdot q_\text{c}}\\[5px]
\end{align}
In the case of condensation, again one speaks of latent heat, since the heat to be released during condensation is not directly evidenced by a change in temperature (from the Latin word “latere”, which means “to be hidden” or “not to appear directly”). In the case of water, the latent heat to be released during condensation is 2257 kJ per kilogram. This is more than five times the amount of heat that would have been required to heat the water from 0 °C to 100 °C! This explains, for example, why steam burns are more dangerous than water burns.
The process of releasing the heat of condensation
Whereas in the case of vaporization one usually has a very clear idea of the process of the transfer of heat, one sometimes has a little difficulty with the releasing of heat in the case of condensation. If, for example, water is vaporized in a pot, then the process of transferring heat takes place actively, so to speak, by heating on a hotplate. If, on the other hand, the gaseous water condenses on a glass plate, for example, then this process does not appear to have been actively forced. It seems as if the process would run by itself, without the need to actively dissipate heat.
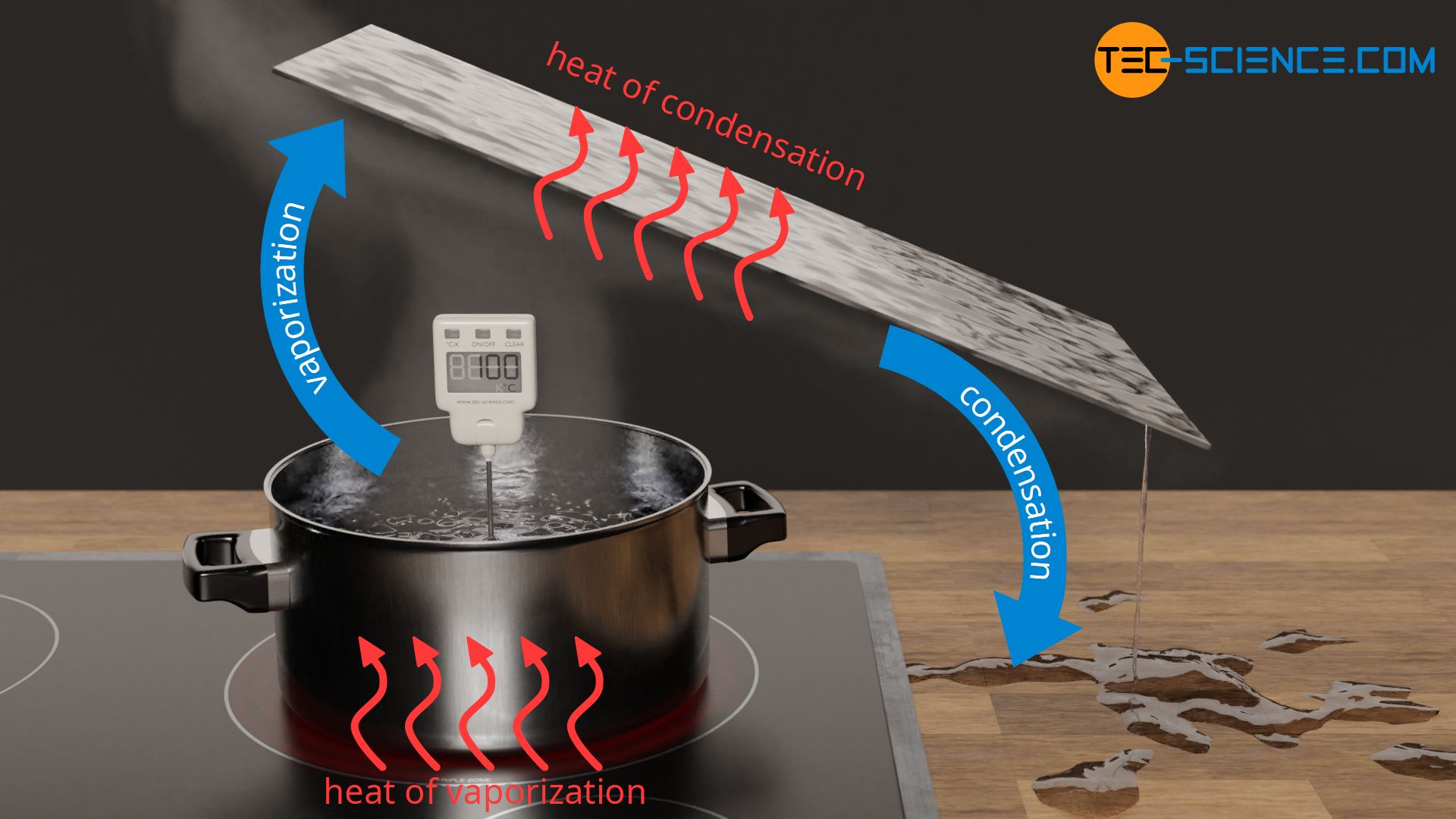
In fact, however, heat must also be released actively in the process. In this case, this is done by the cooler glass plate, which absorbs the heat of condensation and thus dissipates it to the surroundings. In the same way as the hotplate plate actively transfers heat (of vaporization) to the water, the cold glass plate also actively absorbs heat (of condensation).
If, for example, the glass plate had the same temperature of 100 °C as the water vapor, then no more water would condense in this case either, since there would be no heat flow from the vapor to the glass, i.e. no absorption of heat. Conversely, if the hotplate had the same temperature of 100 °C as the liquid water, then vaporization would not occur in this case either, since there is no temperature difference to drive the heat flow.






